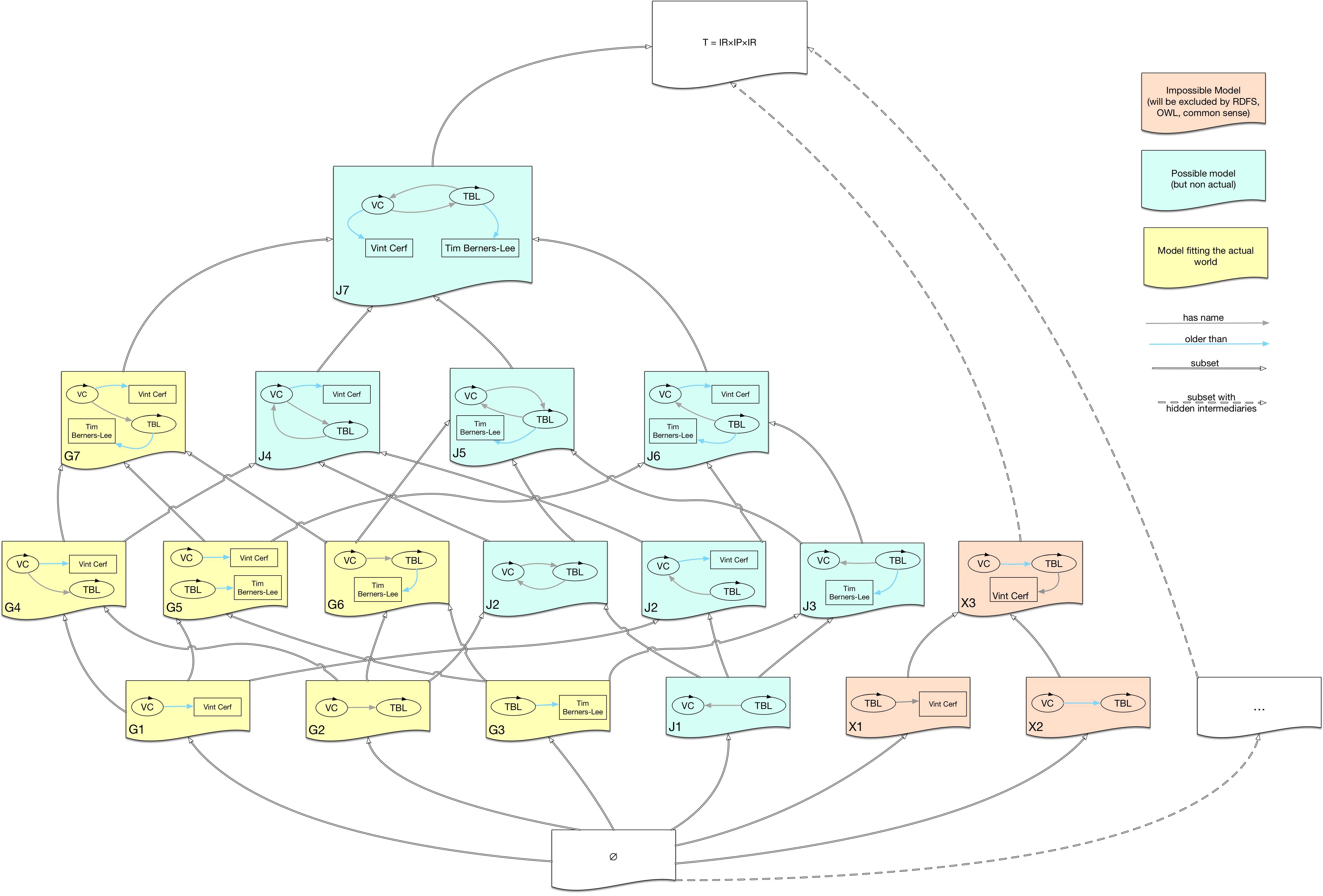
- a category of $Sig$ of Signatures $\Sigma$: For our example we could have a ground graph signature $\Sigma$ containing only a small subset of IRIs and some literals to correspond to the objects in our model. (No existential quantifiers (aka blank nodes) for example). IRIs are just the web equivalent of mathematical symbols, designed so that people can globally coin new symbols without fearing a name clash. They stand for International Resource Identifiers and are specified syntactically by RFC3987RFC3987. IRI ⊇ URI ⊇ URL ∪ URN
- a functor $Mod$ from $Sig^{op}$ to $Cat$ the category of categories selecting for each object in the category $\vert Sig \vert$ a category of models. So I guess that a signature with 4 IRIs and 2 literals can give us a signature to the category of Partially ordered models I drew above.
- a functor $Sen$ from $Sig$ to sets of Sentences in $Set$ (here we need no ur-elements I think, since we can encode the Unicode alphabet with the empty set and sets thereof)
- A relation $\models_\Sigma \space \subseteq \vert Mod(\Sigma) \vert \times Sen(\Sigma)$
replaced https://tools.ietf.org/html/rfc with https://www.rfc-editor.org/rfc/rfc
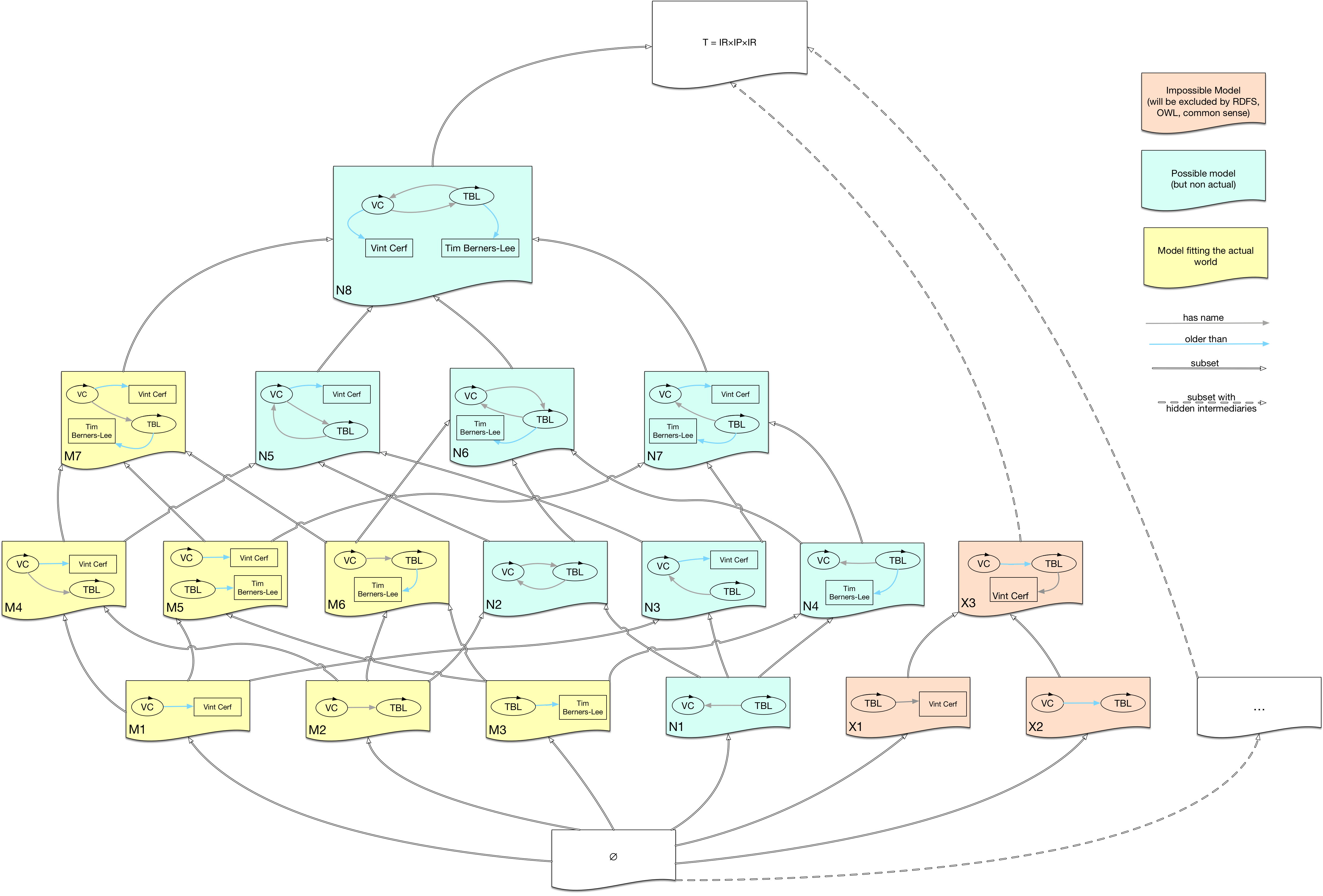
rename the post names with M rather than G, since M stands for models, which is what we are looking at.
Henry Story
- 595
- 2
- 13
text was a bit long, so I moved question in short to the top, and left explanation for after that.
Henry Story
- 595
- 2
- 13
Added link to excellent article on Institution theory https://www.iep.utm.edu/insti-th/
Henry Story
- 595
- 2
- 13
Henry Story
- 595
- 2
- 13