Counting the number of thick regions which overlap a square

Question

Let $S$ be a unit square. As a function of $\beta$, what is the maximum number of $\beta$-fat pairwise-disjoint regions with diameter at least 1 which can intersect $S$?

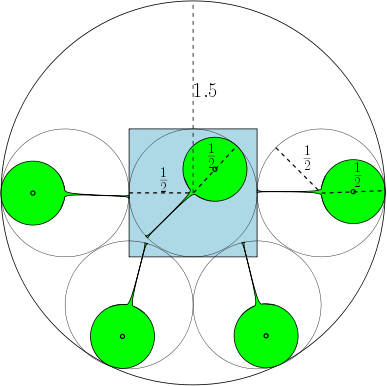
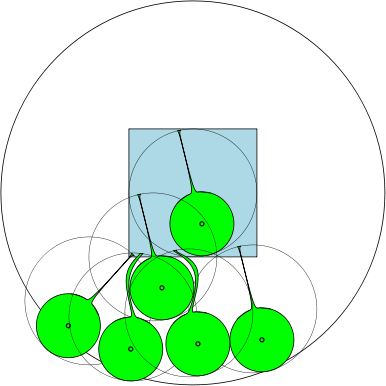
Below, we give a figure showing that for $\beta=1$, the maximum number is 7. What about for $\beta = 2, 3, \ldots, n$?

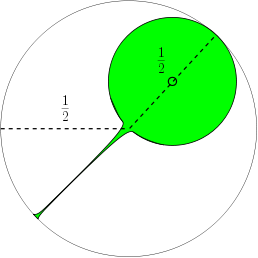
Recall the definition of fat for regions in the plane. Given a region $R$, let circle $C_1$ of radius $r_1$ be the largest circle contained in $R$, and let circle $C_2$ of radius $r_2$ be the smallest circle that contains $R$. The fatness of $R$ is given by $\frac{r_2}{r_1}$, and we say that $R$ is $\beta$-fat, for $\beta = \frac{r_2}{r_1}$.

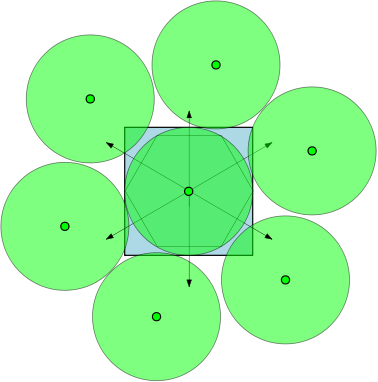
For example, if $r_2 = r_1=\frac{1}{2}$, then the regions are unit circles, and there are at 7 circles with diameter at least 1 which can overlap $S$ without overlapping each other. In the figure below, we have depicted a unit square and 7 unit circles which overlap the square.

Answer 1

I think that the maximum number of pairwise disjoint fat regions which overlap the square should be strongly related to circle packing.

The worst-case shape for a region is something like a "ball & chain". Below I have depicted such a region for $\beta=2$ with diameter 1

.

and these can pack within distance 1 of the unit square obviously much more tightly than I've depicted them.

Note that the actual ball & chain region is defined by the green area, and the outer circle is just a guide to depict the fact that these regions have fatness 2. In fact, the chain part of the region, can "bend" to allow more regions to be packed.